Відео

👍

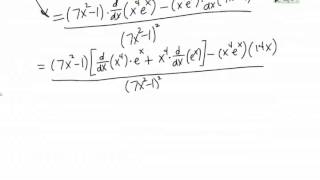
Thank you so much! You helped me get an A on my math test

Heather please come back the people need you

Tomorrow I am going for a debate competition thank you so much for your help

Casimer Knolls

Estella Throughway

Angelina Prairie

Gibson Springs

Bartell Well

Fredy Brooks

that is not the orthogonal projection

Really helpful. Btw we can also use double integration by considering limits of x as well as y to calculate area. is not it?

Not clear concept

You do the humpty hump oddly

Ty do much

Really helpful, thanks teach!

How did you find the y point

These are really good. Thank you!

I think it is important to note that the problem itself doesn't forbid negative x values. Intuitively it is correct, but the math should match. Even the original equation for T is correct for negative x values. The reason for the negative x solution at the end is due to the squaring operation at 14:41. That operation "adds" a solution. Note that the equation before squaring can only work with a non-negative x value (otherwise you get a negative square root). It is a common problem to accidentally add extra solutions to algebra problems this way and worth learning to look out for.

Brilliant! Thank you Heather.

The person who posted the comment 5 years ago probably graduated college by now!! If you see this comment 4 years from now I'll have graduated too!

Thanks for the review, helped remind me of some of the steps I was forgetting.

😇🩷🩷

Thank you so much for your help!! Hopefully I ace my calc test!

Thanks

This is helpful

Who's here because of minecraft?

clearly and helpful even its 9 years ago

ty

So basically you eyeball it

Great explanation!

Wtf

This was super useful and helpful, for finding the exact instantaneous velocity what would be the next step? (The calculus 2 method). Just curious would be nice to know, great video!

Thank you very much.

i have an exam in 23minutes and u just answered a question ive been searching for answers on, thank u so much

Thank you

It’s stupid that they make z up and down when Y is already represented as up and down on a 2D graph. Why wouldn’t z just be added in as forward and back?

Cali

One of the best explanations I've seen! Appreciate the time taken to explain similar triangles. 9 years later and still better then most videos of the same problem!

Hi, How exactly did you graph the function? I cannot get a parabola...all I get is a flat line at the the origin. Thanks

yer

Audio

Amazing explanation thank you so much

Loved it! Great explanation and conceptualization! Really helped me visualize the concept! Thanks!

Thank you❤

Thank you for a really great video! I'm in calc and the textbook is ancient, verbose, and unnecessarily complicated.

so x axis is left/right y axis is up/down and z axis is out depth.

Can anyone give me the link for this pdf?

Thank you Heather

2022 calc 2 playing catch-up already only a week in